Linear Equation Calculator

Linear equations form the foundation of many mathematical concepts, and solving them is a critical skill in both academic and professional settings. A Linear Equation Calculator is a powerful tool designed to simplify this process, making it accessible and efficient for users of all levels. We explores the concept of linear equations, the features of a linear equation calculator, its applications, and more.

What is a Linear Equation?

A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and a single variable. The defining characteristic is that the highest power of the variable(s) involved is always one. These equations can be represented in various forms, such as:

• Standard Form: ax+b=0
• Slope-Intercept Form: y=mx+b

Linear equations are called “linear” because their graphical representation forms a straight line on a coordinate plane. They are widely used in various fields, including physics, economics, and engineering, to model relationships between quantities.

Features of the Linear Equation Calculator

A Linear Equation Calculator is designed to solve equations quickly and accurately. Its features include:

• Support for Multiple Forms: Solves equations in standard form, slope-intercept form, or general form.
• Single and Multi-Variable Solutions: Handles single-variable equations as well as systems with two or more variables.
• Step-by-Step Solutions: Provides detailed explanations for each step in solving the equation.
• Input Flexibility: Accepts integers, fractions, decimals, and even symbolic expressions.
• Speed and Accuracy: Delivers precise results within seconds.

These features make it an indispensable tool for students, professionals, and anyone working with equations.

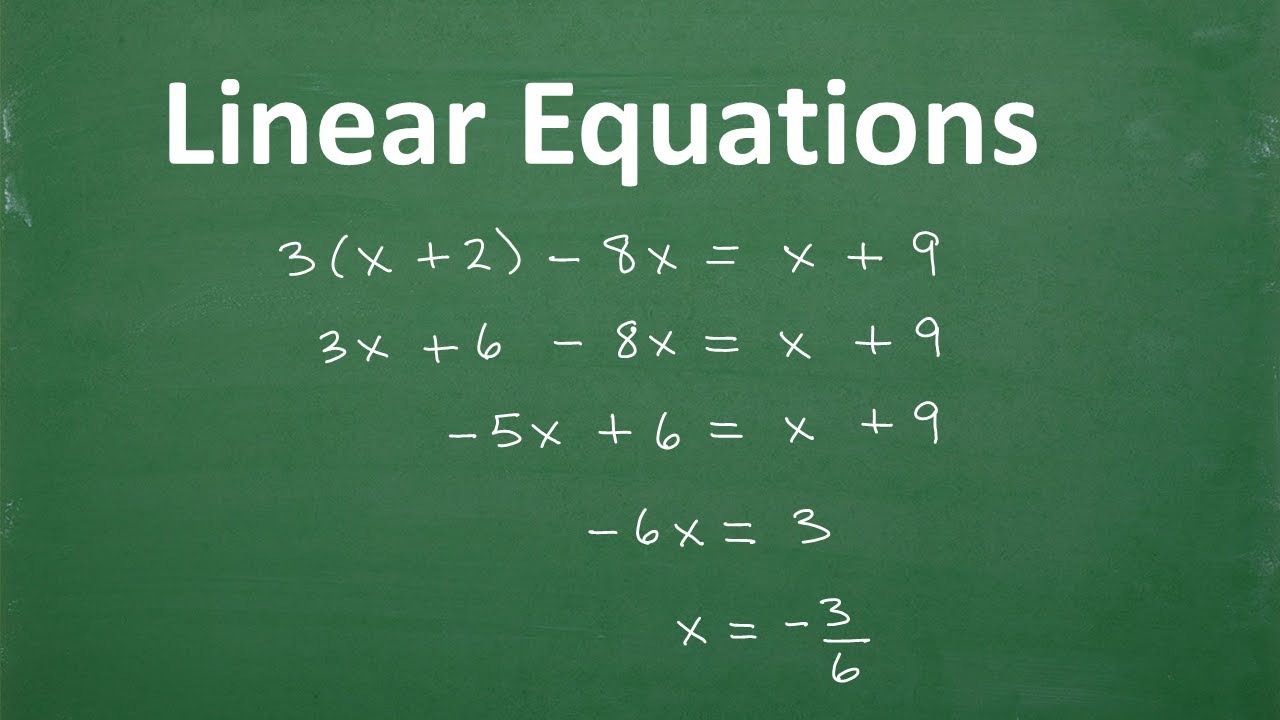
How to Use the Linear Equation Calculator

Using a calculator is a simple and efficient process that allows users to solve equations quickly, regardless of their complexity. Whether you’re a student tackling algebra homework or a professional working on data analysis, this tool streamlines the process. Below is a detailed guide on how to use the calculator effectively.

Inputting the Equation

The first step in solving any linear equation using the calculator is entering the equation correctly. Follow these steps:

1. Open the Calculator Interface: Access the calculator through a website, app, or software tool. Ensure you are using a reliable and accurate platform for your calculations.
2. Enter Coefficients and Constants: Input the numerical values for the coefficients and constants in your equation. 
3. Double-Check Your Inputs: Verify that all inputs are correct before proceeding. Even a small error in entering coefficients or constants can lead to incorrect results.
4. Submit the Equation: Click on the “Solve” or equivalent button to process your input and generate the solution.

Understanding the Results

Once you’ve entered your equation and submitted it, the calculator will analyze your input and provide detailed results. Here’s what you can expect:

1. Solution Output: The calculator will display the value of x.
   • Example: For 2x+3=7, it will show: $x=(7−3)\over2=2$
2. Step-by-Step Explanation: The calculator often breaks down how it arrived at the solution, showing each step in detail. This feature is particularly helpful for students who want to understand the process.
3. Additional Outputs: Depending on the complexity of your input, some calculators may provide:
   • The slope and intercept of a line.
   • The determinant (for systems of equations involving matrices).
   • Warnings about special cases, such as parallel lines (no solution) or coinciding lines (infinite solutions).

Why This Process is User-Friendly

The intuitive design of calculator ensures that even users with minimal algebra experience can navigate them easily. Features like clear input fields, labeled coefficients, and instant feedback make solving equations seamless. Additionally, advanced features such as graphical outputs and step-by-step explanations cater to both beginners and advanced users.

Types of Linear Equations Supported

The calculator supports various types of linear equations:

Single Variable Equations (e.g., ax+b=0)

These are basic linear equations involving one variable. For example:

• 3x+5=0
• Solution: Subtract 5 from both sides (3x=−5), then divide by 3 ($x=−5\over3$).

Two Variable Equations (e.g., ax+by=c)

These involve two variables and are often solved as systems. For example:

• System: x+y=5, x−y=1
• Solution: Add or subtract equations to eliminate one variable and solve for the other.

The calculator can handle systems with two or more variables efficiently.

Benefits of Using a Linear Equation Calculator

Using a linear equation calculator offers several advantages:

1. Time-Saving: Solves complex problems instantly.
2. Accuracy: Minimizes errors in calculations.
3. Learning Aid: Provides step-by-step solutions to enhance understanding.
4. Versatility: Supports multiple types of equations.

These benefits make it an essential tool for anyone dealing with algebraic problems.

Limitations of the Calculator

While highly effective, there are some limitations:

1. Dependency on Input Accuracy: Incorrect inputs yield incorrect results.
2. Limited Contextual Understanding: Cannot interpret word problems directly; users must convert them into mathematical form first.
3. No Conceptual Learning: While it provides solutions, users may not fully grasp underlying concepts without additional study.

Applications of the Calculator

The linear equation calculator is an incredibly versatile tool that finds practical use in a wide range of scenarios. Its ability to solve equations quickly and accurately makes it invaluable for both academic and professional purposes. Let’s explore how this tool is applied in different contexts.

Academic Use

In the academic world, linear equations are a cornerstone of algebra and mathematics education. Students often encounter these equations in homework, exams, and projects, making the linear equation calculator a vital resource for learning and problem-solving.

One of its primary uses in academics is solving homework problems. Students frequently deal with equations that require precise calculations, and the calculator simplifies this process by providing instant solutions.

Finally, the calculator is an excellent tool for exam preparation. By practicing with various types of linear equations and reviewing detailed solutions, students can build confidence and improve their problem-solving speed. 

Professional Use

Outside of academia, the linear equation calculator is widely used in professional settings where mathematical modeling and analysis are essential. Its ability to handle complex systems of equations makes it a valuable tool across multiple industries.

In engineering, for instance, professionals rely on linear equations to model relationships between variables in structural designs, electrical circuits, and mechanical systems. Linear equations are frequently used to model these relationships, enabling economists to make predictions and inform decision-making processes. 

Economists also benefit from this tool when analyzing relationships between economic variables such as supply and demand or cost and revenue. 

In the field of data science, linear equations play a crucial role in regression analysis—a statistical method used to identify relationships between variables. Data scientists use regression models to predict outcomes, optimize processes, or uncover patterns in large datasets. 

Other professionals who benefit from this tool include financial analysts calculating break-even points, architects designing structures based on spatial relationships, and researchers modeling scientific phenomena using linear approximations. In each case, the calculator enhances productivity by automating tedious calculations and providing reliable results.

Bridging Academic and Professional Applications

What makes the linear equation calculator truly remarkable is its ability to bridge academic learning with real-world applications. Students who become proficient with this tool during their studies often find it equally useful when they enter the workforce. By mastering its features early on, they gain a competitive edge in fields where mathematical problem-solving is essential.

Conclusion

A Linear Equation Calculator is an invaluable tool for solving algebraic problems efficiently and accurately. By supporting various types and providing detailed solutions, it serves both academic and professional purposes effectively. However, users should complement its use with manual practice to strengthen their understanding.

FAQ